Today’s Wonder of the Day was inspired by Ryan. Ryan Wonders, “What is the Mobius Strip?” Thanks for WONDERing with us, Ryan!
Have you ever heard about infinity? If so, you might know the symbol for infinity. It’s called the lemniscate, which means “ribbon.” Did you know that the lemniscate looks a lot like another mathematical shape? It’s true! Drumroll please . . . Wonder Friends, meet the Möbius strip!
The Möbius strip is named after mathematician and astronomer August Ferdinand Möbius. He came up with the idea in September 1858. German mathematician Johann Benedict Listing independently thought of the same idea in July 1858. Unfortunately for Listing, one of the most famous surfaces in mathematics was named for Möbius, not Listing.
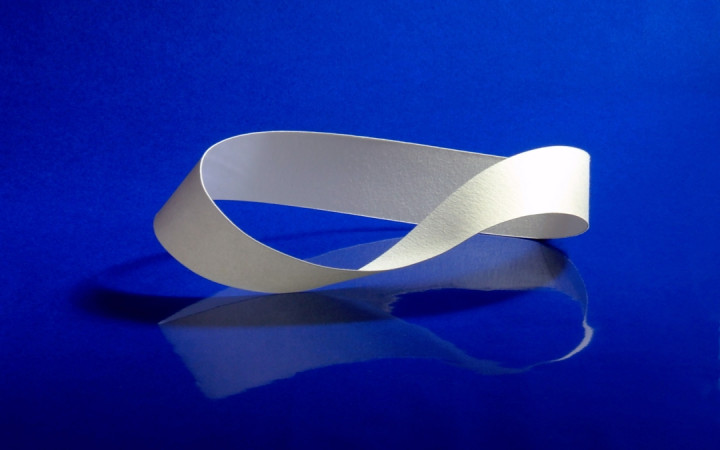
So what’s the big deal with the Möbius strip? It’s quite simple, actually. The Mobius strip is famous because it has only one side and one edge.
Can you picture what we’re talking about? It helps to make your own Möbius strip (see today’s Try It Out! section for instructions). Cut a long strip of paper. Then put a half twist in it, and glue or tape the ends together.
While the original piece of paper clearly had two sides, the Möbius strip you made has just one. Don’t believe us? Try drawing a line on both “sides” of the Möbius strip without picking up your pencil. Can’t do it, can you?
It might also help you to imagine ants walking along the Möbius strip. Have you ever seen M.C. Escher’s famous picture, Möbius Strip II (Red Ants)? It showed that ants could walk on a Möbius strip indefinitely!
Maybe that’s why people connect the Möbius strip to the idea of infinity. It may also be why the lemniscate symbol for infinity resembles a Möbius strip!
You won’t just find the Möbius strip used in math class! For example, conveyor belts have been designed as giant Möbius strips. Why? It helps them last longer. The entire surface area gets the same amount of wear and tear. The Möbius strip has been used in similar ways in recording tapes and printer ribbons.
The Möbius strip is one of the most curious shapes in mathematics. Can you think of any other ways to use it? It may seem like a pretty complicated idea. But just like anything else, you’ll understand the Möbius strip better the more you use it!
Standards: CCRA.L.3, CCRA.L.6, CCRA.R.1, CCRA.R.2, CCRA.R.4, CCRA.R.10, CCRA.SL.1